Locating birds by their calls with improvised equipment. Can algorithms rescue this setup?

Question

In winter, I can usually hear one or two birds in an area, and I occasionally see them flitting through the air – but it's usually too dark to see them except in motion, silhouetted against the sky. This winter, I want to see if I can pinpoint some noisy birds without waiting for them to move, and hopefully photograph them.

I can place four microphones of varying quality in a sort of irregular tetrahedron shape, three in boxes on the ground and one on a deciduous tree. The microphones aren't very directional, but I can calibrate their frequency response.

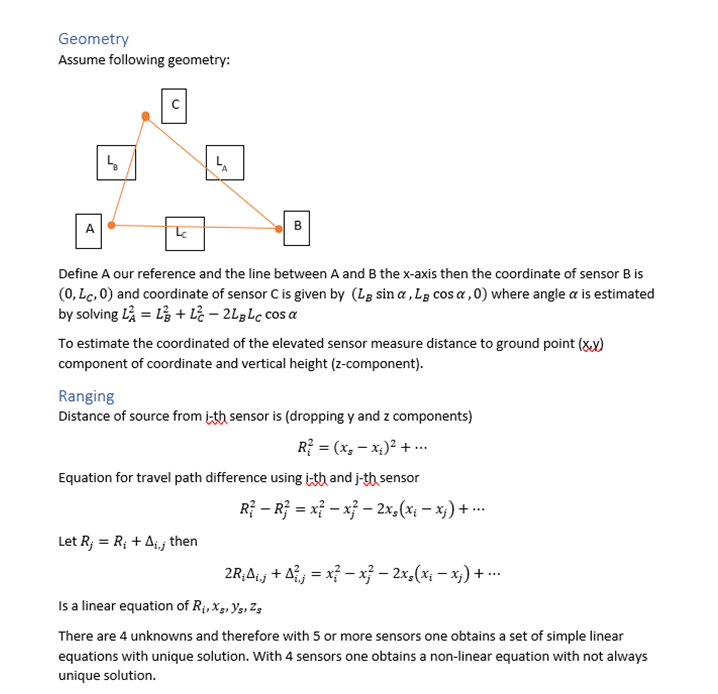
Assuming I've got nearly-synchronised feeds from these microphones, how could I use this data to identify the source location of the sound? My basic idea would be to use the timing and loudness data to construct two sets of four spheres, then see where they all approximately intersect. The wind in winter can affect sound propagation enough for me to hear it, which I expect would throw this off. There would also be echoes off the ground, and nearby buildings, which I don't know how to compensate for. I've heard of tricks related to frequency-dependent attenuation, but judging by how hard I find it to locate birdsong with my two ears and brain, I don't think this works well with chirping sounds.

I can add a couple more mics (repurposed headphone speakers, so lower-quality), a digital thermometer, and possibly a crude (handmade, indirectly-calibrated) anemometer to the setup, if it'd help.

Answer 1

First I would add a 5th microphone, so that the maths are simpler. (edit: what are the minimum number of micro/hydro-phones needed to perform 3d tracking?)

I would measure (as precise as you can; e.g. with a laser) the separation between each pair of microphones and the distance from multiple reference points.

Then we need a little bit of maths to solve the multiple measurements together with the dime delays into a source location.

Traditionally, if the locations are known as x,y,z then maths is straight forward and discussed also in this SE. The interesting aspect is to use measured microphone distances to estimate also microphone locations. I have not done that yet but may try.

Edit: it all translates to triangular geometry

Edit2: here comes a basic outline of the procedure

Answer 2

Sounds like a neat system, all the best!!

To localise sounds, you need the time-difference-of-arrivals across channels. If your direct path is the strongest, then normally simple cross-correlations are enough, the peaks can then be used to find the 3d position of the birds. The math required to the positions are in the answers to the question pointed out by @WMXZ.

I wouldn't really bother with temperature, humidity and other such weather measurements. Yes, sure it will affect localisation through the speed of sound, but I've done this broad comparison of with and without detailed weather data and remember at most 1-5% range difference.

The attenuation of sound for birdsong type frequencies is almost negligible over short distances (esp. in comparison to attenuation for ultrasound). For a more detailed reference on weather conditions' effects on sound speed and attenuation check out Goerlitz 2018 (link)

Answer 3

To address one of your side questions re: precise localization using a microphone dangling from a tree. You can take efforts to minimize movement of this microphone. If suspended by a rope, you can consider:

• Attach rope onto something more stiff to minimize movement (such as a stick, stiff garden hose)
• Have > 1 attachment point ('anchored' by 3 attachment points)
• Have a small weight attached to the bottom so that it requires more force to move it

You can also measure the amount of movement using an accelerometer.

[I also wonder if you can attach a phone to the recorder and use the internal accelerometer to measure the amount of movement of the recorder]