How do you know when minimum noise measurements are due to the instrument's noise floor or due to the environment?

Question

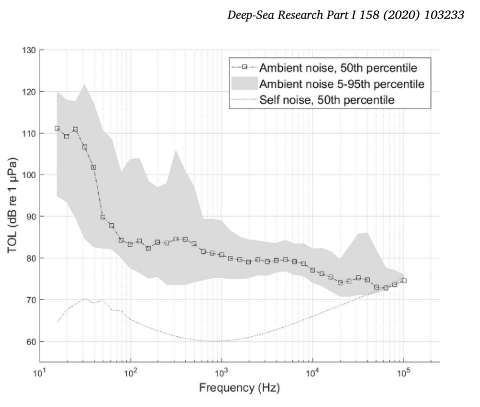
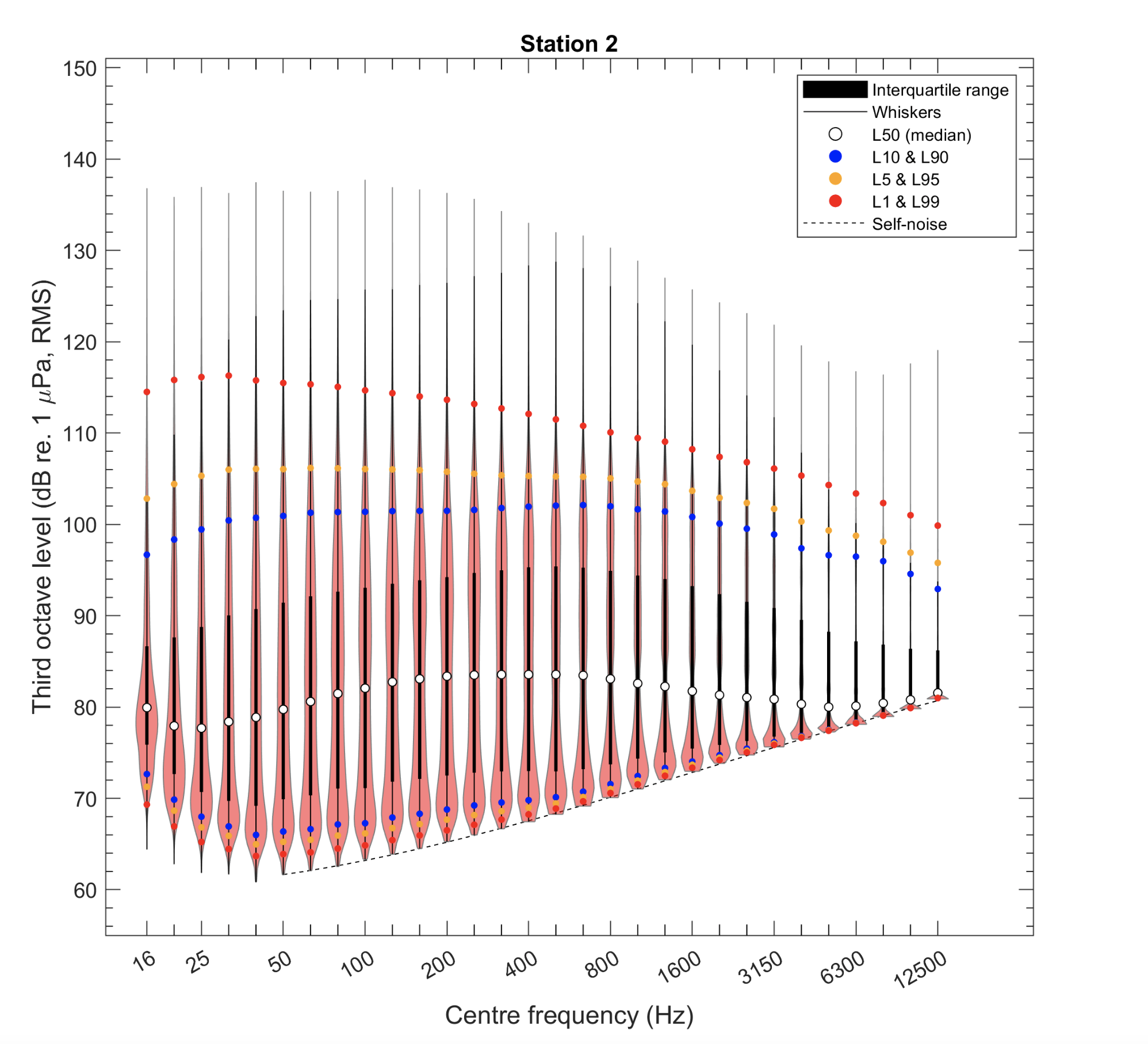
I have some ambient noise recordings and am uncertain if the lower SPLs represent true low noise levels in the environment, or are perhaps due to the noise floor of the instrument itself.

I have plotted the third octave levels of an ambient noise recording, but am noticing that the minimum sound pressure level values increase at high frequencies where I don't expect them to. I suspect that this is a reflection of the sensitivity of my instrument, rather than a true representation of ambient noise in the environment.

I have seen some examples in the literature (see attached) of plotting the "self noise" of an instrument on top of this, however I am uncertain how to get these values across the frequency range of my instrument. How do I determine what this self noise (or 'noise floor') is, so I can plot it atop mine too? I want to get this across the spectrum of the recording range of an acoustic monitoring instrument.

EDIT: Device is a SoundTrap, so I cannot disassemble the recording chain.

Answer 1

It's not the gold standard, but you can sometimes get a good idea of the self noise of an instrument by looking at the distribution of noise values. Take a look at this image from the supplementary material of;

Ladegaard, M., Macaulay, J., Simon, M. et al. Soundscape and ambient noise levels of the Arctic waters around Greenland. Sci Rep 11, 23360 (2021). https://doi.org/10.1038/s41598-021-02255-6

Long term noise measurements will usually form some sort of Gaussian like distribution - in this figure the noise values at lower frequency are higher amplitude and form the expected distribution. However, at higher frequencies the noise distributions begin to have a "hard edge" at the lower bound. This is almost certainly because the amplitude has fallen below the noise floor of the instrument (and so lower amplitude noise measurements are registered as the self noise of the instrument instead). Interpolating these lower bounds (dashed line) can provide an estimate of the noise floor of the instrument.

Answer 2

I suggest you take the instrument that you used to make recordings in the wild, and make recordings in a quiet room, ideally one padded with foam - like those used for bat echolocation research - with the lights out (as lights have a hum that can contaminate your recordings). Then run your TOL analysis on this data, and plot whatever percentile of this atop the TOL percentiles of your ambient data. In the figure you attached, we can see that above ~30 kHz, the ambient noise recordings are limited by the self noise of the instrument. This means that above this frequency, your recording chain is not able to capture how quiet the environment is.

Answer 3

I would remove the micro/hydrophone and replace it with a small resistors. what you then measure is the thermal noise of the resistor.

If you change the resistor value, you can measure in addition to the electronic noise also the gain of the acquisition chain

The impact of the resistor R on the measured voltage V_out is

V_out^2=(e_n^2+i_n^2 * R^2 + 4 * k_B T * R) * G^2

where

e_n input noise voltage; i_n input noise current; G gain

For R=0 (short) we have V_0^2=(e_n^2 ) * G^2

which gives us an expression for Gain G

In the end we have a set of linear equations for e_n^2, i_n^2

e_n^2 ((V_out^2)/(V_0^2 )-1)-i_n^2 * R^2 = 4k_B T *R

At 20 C (293 K) we have :

4k_B T=0.016173 (nV^2)/(Ω*Hz)

Using at least 2 measurements with R>0 one obtains values for input noise voltage, input noise current, and gain

Edit: this gives self noise for whole acquisition chain.

Edit 2: The expression for 4k_B T is relative 1 Hz. What I typically do is not to measure the broadband noise voltage but the spectral density so may self noise estimates ate also relative to 1 Hz.

Edit 3: In practice, I record data having sensor (hydrophone in my case) replaced with different resistors and use MATLAB's pwelch function to get the PSD.

ceterum censeo: we need latex style math formula!